Melting of electronic/excitonic crystals in 2D semiconductor moir´e patterns: A perspective from the Lindemann criterion

Jiyong Zhou(周纪勇), Jianju Tang(唐剑炬), and Hongyi Yu(俞弘毅),2,†

1Guangdong Provincial Key Laboratory of Quantum Metrology and Sensing&School of Physics and Astronomy,Sun Yat-Sen University(Zhuhai Campus),Zhuhai 519082,China

2State Key Laboratory of Optoelectronic Materials and Technologies,Sun Yat-Sen University,Guangzhou 510275,China

Keywords: moir´e pattern, transition metal dichalcogenides, electronic crystal, excitonic crystal, Lindemann criterion

The formation of long-wavelength moir´e patterns in van der Waals stacking of two-dimensional (2D) semiconducting transition metal dichalcogenides (TMDs) has introduced a new platform for studying exotic quantum phenomena.[1,2]In the past few years,experiments have detected various electronic correlated insulators[3-15]and moir´e confined interlayer excitons[16-19]in these systems, which come from the enhanced Coulomb interaction by the 2D geometry combined with the presence of a moir´e superlattice potential.By continuously tuning the doping density of the bilayer TMDs moir´e system,a variety of quantum electronic crystals with different lattice types have been detected under low temperatures.Besides the triangular Mott insulators at a filling factor of one electron per moir´e supercell (ν=1), the generalized Wigner crystals and stripe crystals under fractional fillingsν ＜1 as well as the monolayer and bilayer Wigner crystals with the absence of moir´e patterns have also been observed,[4,8,9,11,13,15]signifying the long-range nature of the Coulomb interaction.Meanwhile in a bilayer system, an electron-hole pair in opposite layers can bound into an interlayer exciton (IX)which has a permanent electric dipole perpendicular to the 2D plane.[20]The dipolar repulsion between IXs trapped in different moir´e potential minima can give rise to the formation of a quantum excitonic crystal,[21-23]as being observed in recent experiments.[24-27]

The phase transition between the crystal and liquid comes from the competition between the kinetic and potential energies,which favor delocalization and localization,respectively.A melting of the crystal will happen when the fluctuation of the crystal site becomes sufficiently large compared to the inter-site distance, which can be realized by the increase of the temperatureT(thermal melting) or the decrease of the inter-site distance (quantum melting).This leads to the traditional Lindemann criterion,which states that the melting occurs when the Lindemann ratioexceeds some critical valueηc.Here〈r2〉 is the mean-square displacement of the crystal site from both the quantum and thermal fluctuations, andλis the distance between the nearest-neighbor sites.The traditional Lindemann criterion is rather successful in describing the melting of 3D atomic crystals (withηc≈0.1).Meanwhile numerical analyses have shown that it also applies to the quantum melting of 2D quantum crystals atT=0 (withηcbetween 0.2 to 0.25).[28-30]However,it fails to describe the thermal melting of 2D crystals,as〈r2〉diverges logarithmically with the area of the 2D system whenT ＞0.Alternatively, one can consider the fluctuation of the relative displacement〈(rn-rn＇)2〉between a pair of nearestneighbor sites (n,n＇), and define a modified Lindemann ratiowhich is finite underT ＞0.[31]The modified Lindemann criterion states that the crystal melting occurs whenη(m)exceeds some critical value≈0.31 for 2D quantum crystals formed by the Coulomb or dipolar repulsions.[32]It should be emphasized that although the Lindemann criterion has been confirmed by numerical simulations and experiments,the obtained empirical constantsηcandη(m)cfrom different literatures have slight variations.Nevertheless,the melting process can be qualitatively analyzed from the dependences ofηandη(m)with system parameters.In this work,we calculateηandη(m)to get some perspective about the quantum and thermal melting of the electronic/excitonic crystals recently discovered in bilayer TMDs moir´e patterns.

Throughout the paper we stick to the conventione=¯h=4πε0= 1, withethe charge of an electron, ¯hthe reduced Planck constant andε0the vacuum permittivity.The energy scale that characterizes the crystal melting corresponds to the inter-site interaction strength.In 2D layered materials,the interaction between electrons corresponds to the Coulomb potential modified by the atomically-thin geometry of the layered structure,which can be expressed in the Rytova-Keldysh form[33]

whereH0andY0are the Struve and 2nd-kind Bessel functions, respectively.r0is the 2D screening length (largerr0implies stronger screening effect of the layered material),andεis the relative dielectric constant of the environment.Different monolayer TMDs have similar screening lengthsr0≈5/εnm,[34]whiler0≈10/εnm can approximately describe Coulomb interactions in bilayer TMDs.In the limitr0→0,VC(r)→1/(εr).Meanwhile, the interaction between IXs in bilayer structures is given by the electric dipolar repulsionVD(r)=2Vintra(r)-2Vinter(r), whereVintra(-Vinter) corresponds to the intralayer Coulomb repulsion between two electrons or two holes(interlayer Coulomb attraction between an electron and a hole).Here for simplicity,we have modeled IXs as point dipoles because of the small Bohr radius(≈2 nm[35]).Modified by the 2D geometry of the bilayer structure, the Fourier transforms ofVintra(r)andVinter(r)have the following forms:[36]

UnderT=0, we use two approaches to obtain〈r2〉 and the traditional Lindemann ratio of the triangular crystal.The first approach is a mean-field treatment on the interaction between crystal sites,which can give an intuitive picture for the localization of the particle.Near a lattice site located atR0=0,a particle feels other particles’ repulsion as well as the background moir´e potential (see the illustration in Fig.1(a)), the mean-field trapping potential can be written as

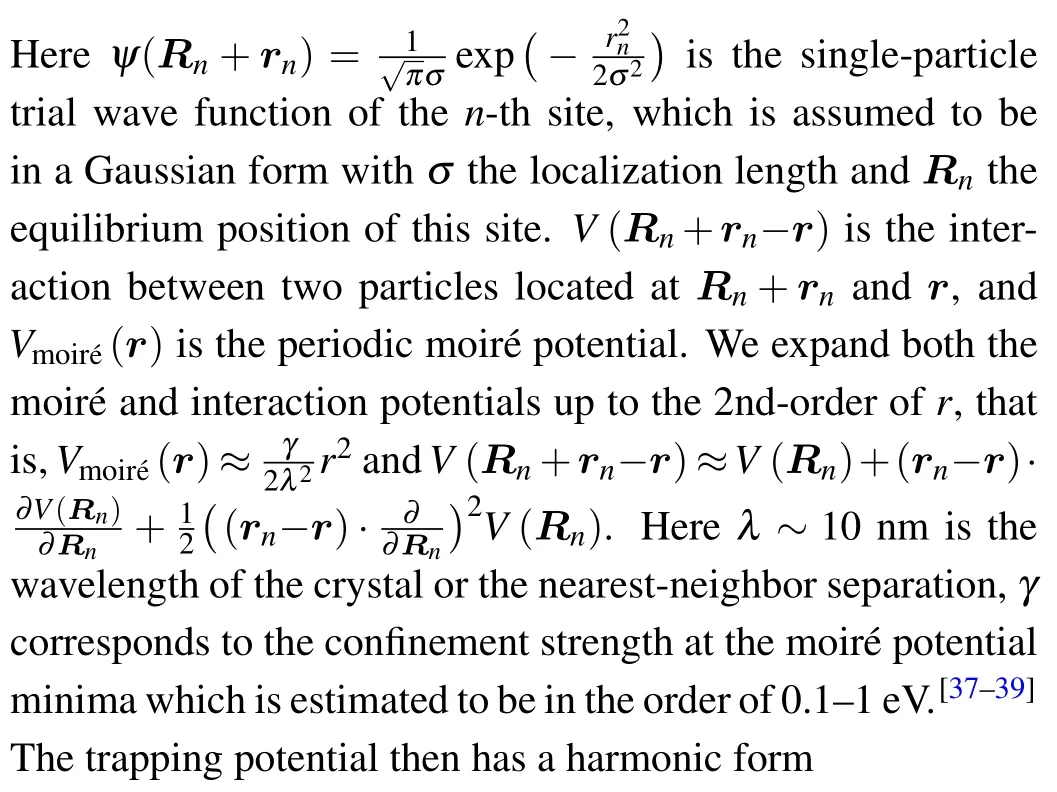
This results in

This gives a rigorous expression for the mean-squaredisplacement

In obtaining Eqs.(6)and(8),we have used the harmonic approximation which requires the hopping between moir´e potential minima to be suppressed by the interaction, that is,the strong correlation limit.On the other hand, a weak correlation limit can be realized in TMDs moir´e patterns under a filling factorν ≪1, where the distance between the crystal sites is much larger than that between the nearest-neighbor moir´e potential minima.In this case,the hopping from an occupied moir´e potential minimum to an empty one costs very small interaction potential thus cannot be ignored.This implies that each electron/exciton site will cover multiple moir´e potential minima, resulting in〈r2〉 significantly larger than those in Eqs.(6) and (8).To calculate the correct〈r2〉 value in the weak correlation limit, one can first solve the singleparticle mini-bands under the periodic moir´e potential to obtain the effective massmmoir´eat the energy minimum.Then the electrons/excitons are treated as particles with massmmoir´ewhich weakly interact with each other, and Eqs.(6) and (8)withγ=0 can be used to get the Lindemann ratio.However,the inevitable disorder potential will dominate over the interaction underν ≪1,which introduces fluctuations and can break the long-range crystalline order.[41,42]Below we focus on the strong correlation limit,and assume that the harmonic approximation is always valid.

For the electronic crystal withV(r)=VC(r) (Eq.(1)),we show our calculatedηas a function of the moir´e wavelengthλin Figs.1(b)-1(d), for several values of environmental screeningε, screening lengthr0and moir´e trapping strengthγ.Unless specified,we set the electron effective mass asm=0.5m0withm0the free electron mass.The results obtained from Eqs.(6) and (8) are shown as solid and empty symbols,respectively,which show qualitative agreement.The rigorous results ofηfrom the phonon dispersion are generally larger than the mean-field results(especially whenγ=0),implying that the inter-site correlation not taken into account in the mean-field treatment can lead to delocalization.[43]Figures 1(b)and 1(c)correspond to the case of a suspended TMDs layer (ε=1), whereas Fig.1(d) simulates a structure encapsulated by thick hBN layers (ε=5).Note thatm=0.8m0is close to the measured effective mass ofK-valley electrons in monolayer MoSe2.[44]Just as expected,largerr0values lead to stronger 2D screening thus weaker localization (or largerη).For a givenr0value,ηdecays with the increase ofλ,implying that the crystal phase is favored under a low electron density.This can be understood from the mean-field result in Eq.(6):for large values ofλ,ηscales asλ-1/4whenγ=0,but scales asλ-1/2for a finiteγ.According to the Lindemann criterion,the electronic crystal will melt into a liquid whenηis aboveηc≈0.2-0.25.[28,29]From Figs.1(b)-1(d), we can see that a strong moir´e confinementγcan greatly facilitate the formation of the crystal phase with a short wavelengthλ～10 nm,especially when the environmental screeningεis large(Fig.1(d)).

For the excitonic crystal,we show the calculated interaction strengthV(r)=VD(r)as a function ofrin Fig.2(a)underd=0.6 nm and several values ofr0.Unlike the Coulomb interaction between electrons whose strength is always weakened by increasingr0, the dipolar interaction between IXs shows a complicated behavior withr0.As shown in Fig.2(a),the strength ofVD(r) gets enhanced (weakened) by the 2D screening whenr～r0(r ≪r0).As will be shown below,such an enhancement can facilitate the formation of the crystal phase.Forr ≫r0,the dipolar interaction converges to the traditional formVD(r)≈d2/r3in 3D homogeneous space.

Fig.2.(a) The dipolar interaction strength between two IXs under ε =1 and d =0.6 nm for three different r0 values.The inset illustrates the intralayer(interlayer)Coulomb interaction Vintra (-Vinter)between the same charge(opposite charges).(b)-(d)The calculated Lindemann ratio as a function of λ,under different sets of parameters.The exciton effective mass is set as m=m0.The solid and empty symbols are the results obtained from the mean-field treatment(Eq.(6))and the phonon dispersion(Eq.(8)),respectively.The insets show the overlap between nearest-neighbor wavepackets for the chosen data points.

The calculated traditional Lindemann ratio for the excitonic crystal with an IX effective massm=m0is shown in Figs.2(b)-2(d), where the solid (empty) symbols correspond to results obtained from Eq.(6)(Eq.(8)).For the case ofγ=0 andr0=0(red line in Fig.2(b)),ηbecomes larger when increasingλ,implying that the crystal phase is favored under a smallλ.However the obtained value ofηis quite large(especially the rigorous results from Eq.(8)which are significantly larger than the mean-field results),implying that a rather high IX density is needed to realize the crystal phase.However,when taking into account the finite Bohr radius(≈2 nm),excitons will undergo a Mott transition to the electron-hole plasma above a critical density,thus preventing the crystal phase from forming under this set of parameters.Meanwhile, a finiter0can facilitate the formation of the crystal phase,as indicated by the very different behaviors of ther0=0 andr0/=0 curves in Fig.2(b).Forγ=0 andr0/=0,ηshows an anomalous behavior of first decreasing then slowly increasing withλ,exhibiting a minimum at a certainλvalue.Such a behavior is due to the fact that the dipolar interaction strength gets enhanced by the 2D screening in the regimeλ～r0(see Fig.2(a)).The presence of a finite moir´e confinementγcan further facilitate the formation of the crystal phase.In Fig.2(c) withγ=0.2 eV,ηbecomes smaller when increasingλ, similar to that in the electronic crystal.Now the crystal phase withη ＜ηccan be realized whenλis sufficiently large.Figure 2(d)corresponds to a large environmental screening(ε=5,r0=1 nm),in this case a largeγis essential for realizing the crystal phase.

We now consider the thermal melting of the electronic/excitonic crystal.In the absence of the moir´e confinement (γ= 0), the long-wavelength transverse phonon mode has a linear dispersionωT,k→0=ckwithcthe group

Fig.3.(a)Schematic illustration of the triangular electronic crystal under ν=1 and its Brillouin zone.(b)The contour plots of η(m)with λ and T for triangular electronic crystals,under various system parameters.(c)The linear-stripe electronic crystal under ν=1/2 and its Brillouin zone.(d)The contour plots of η(m)for linear-stripe crystals.(e)The zigzag-stripe electronic crystal under ν =1/2 and its Brillouin zone.(f)The contour plots of η(m) for zigzag-stripe crystals.(g)The honeycomb electronic crystal under ν =2/3 and its Brillouin zone.(h)The contour plots of η(m) for honeycomb electronic crystals.

Figure 3(a) is the schematic illustration of a triangular electronic crystal,the correspondingη(m)values as functions ofλandTunder different sets of system parameters are shown in Fig.3(b).η(m)increases with the increasing ofTand the decreasing ofλ, and depends sensitively on the moir´e confinement strengthγ.We note that an electronic Wigner crystal with a wavelengthλ ≈27 nm has been observed in hBNencapsulated monolayer MoSe2below 11 K,[10]which is in qualitative agreement with theγ=0 result in Fig.3(b).The quantitative disagreements in the range ofλandTfor the crystal phase could be due to the discrepancy inη(m)cand system parameters likeε,r0andm.For finiteγvalues,the electronic crystal can form atλ～10 nm and below a critical temperature which ranges from several tens Kelvin to above 100 K(depending on parameters likeγ,ε,r0andm).This is also in qualitative agreement with the experiment in Ref.[8], where electronic crystals underν=1 in WSe2/WS2moir´e patterns have been observed below～150 K.We emphasize that the critical valueη(m)cobtained using different method can be different,thus our result ofη(m)can only give a qualitative estimation to the melting temperature.Meanwhile,η(m)cis also temperature-dependent.For instance, in the classical limit such that the thermal fluctuation dominates over the quantum fluctuation (the high-temperature and low-density case), the critical value becomesη(m)c≈0.15.[32]Due to the small effective mass of the electron or IX,here we focus on the limit that the quantum fluctuation dominates over the thermal fluctuation,and ignore the temperature-dependence ofη(m)c.

Electronic crystals other than the triangular type can form under a fractional filling factor, including the linear-stripe(ν= 1/2), zigzag-stripe (ν= 1/2) and honeycomb (ν=2/3) crystals.[4,8,9,11,13,15]We have calculatedη(m)for these electronic crystals using the harmonic approximation, where〈(rn-rn＇)2〉 is obtained from an equation similar to Eq.(9)and the phonon dispersions have been calculated in Ref.[40].Figures 3(c), 3(e), 3(g) are the schematic illustrations of the linear-stripe,zigzag-stripe and honeycomb electronic crystals,respectively,and Figs.3(d),3(f),3(h)show the correspondingη(m)values.Note that these types of electronic crystals are dynamically stable only under a large enoughγ(certain phonon modes have imaginary frequencies under weakγvalues),[40]thus only the results with finiteγare shown.Compared to the triangular crystal, the lower densities of the linear-stripe,zigzag-stripe and honeycomb crystals decrease the Coulomb interaction strength and result in largerη(m)values.We note that in principleη(m)cshould vary with the lattice type.It is found that the honeycomb-type bilayer Wigner crystal observed in MoSe2/hBN/MoSe2heterostructure exhibits a significantly larger modified Lindemann ratio compared toη(m)cof the triangular crystal, where the crystal lattice constant is≈6 nm and two subsites are located in opposite layers separated by 1.6 nm.[45]Nevertheless,we can get some perspective from how the calculatedη(m)depends onλ,Tand other parameters.For the linear-and zigzag-stripe crystals formed underν=1/2,the linear-stripe crystal has a significantly largerη(m)than the zigzag-stripe one under a weakγor smallλ(see Figs.3(d) and 3(f)), implying that the latter can be realized more easily.On the other hand, all lattice types have similarη(m)values for a largeγor largeλ, because in this limit the carrier localization is dominated by the moir´e confinement and the inter-site interaction plays a minor role.We note that in Ref.[8], the electronic crystals under fractional fillings are found to have melting temperatures significantly lower than that underν=1 (～30 K vs.～150 K).Meanwhile for different triangular crystals formed underν=1,1/3,1/4 and 1/7,the corresponding melting temperatures are lower for smallerν.These could be due to the following reasons that are ignored when we use Eq.(9) to calculateη(m): (1)The disorder in the system can suppress the long-range crystalline order,[41]whose effect is significant when the inter-site interaction is weak.(2)Whenνdecreases from 1 to≪1,the hopping between nearest-neighbor moir´e potential minima becomes more and more important, which leads to largerη(m)values than our calculated results.

Figure 4 is about the modified Lindemann ratio of excitonic crystals.Figures 4(a), 4(c), 4(e), 4(g) show our calculated phonon dispersions of the triangular, linear-stripe,zigzag-stripe and honeycomb excitonic crystals, respectively,under the parameters ofε=1,γ=0.2 eV,λ=7 nm,d=6 °A.Due to the enhanced dipolar interaction strength, the variation range of the phonon frequency underr0=5 nm or 10 nm is significantly larger than that underr0= 0 nm.The correspondingη(m)values are shown in Figs.4(b), 4(d), 4(f),4(h) as contour plots.Similar to the electronic crystal, whenγ=0 only the triangular-type excitonic crystal is dynamically stable with real phonon frequencies.In this case,η(m)has its minimum located at some finiteλ.However, min(η(m))is aboveη(m)c≈0.31 unless the dipolar interaction is quite strong (e.g., when the interlayer separationdis large, see Fig.4(b)).This is distinct from the electronic crystal whereη(m)can always decrease to near zero for a large enoughλ(Fig.3(b)).Note that in experiments,most of the observed IXs haved ≈0.6 nm in bilayer TMDs ord ≈1 nm in van der Waals stacked TMDs/hBN/TMDs system,where the dipolar interaction seems not strong enough to realize the excitonic crystal underγ=0.On the other hand,under a finite moir´e confinement (γ ＞0) the behavior ofη(m)for the excitonic crystal is qualitatively similar to that of the electronic crystal.In this case, the excitonic crystal can form under a large enoughλand a low enough temperature.Again, we estimate that the melting occurs at a critical temperature ranging from several tens Kelvin at smallγto above 100 K at largeγ.

We note that in experiments, the excitonic crystals are observed only under integer fillings but not for fractional ones.[24-27]We expect that the formation of an excitonic crystal with a filling factorν ＜1 will be much harder than the electronic crystal,because:(1)The dipolar interaction between excitons is much weaker than the Coulomb interaction between electrons,thus the effect of the disorder is more significant for the exciton case which can destroy the excitonic crystalline order.(2)In theν ≪1 limit,IXs should be modeled as particles underγ=0 but with an effective massmmoir´edetermined from the moir´e mini-band, which weakly interact with each other through the dipolar potential.Figure 3(b) (Fig.4(b)) shows thatη(m)of the electronic (excitonic) crystal decreases (increases) with the increase ofλwhenγ=0 andλis large.Thus in theν ≪1 limit with vanishing disorder,the electronic crystal with a largeλcan always form,whereas the excitonic crystal cannot form.

In summary,we have calculated the traditional and modified Lindemann ratios of electronic/excitonic crystals recently discovered in 2D semiconductor moir´e patterns, which are used to analyze the quantum and thermal melting of these crystals.We find that in the absence of a moir´e potential, the electronic crystal with a wavelength～30 nm or larger can form under a temperature of tens Kelvin,whereas the excitonic crystal is very difficult to form in bilayer TMDs systems.A strong confinement from the moir´e potential can facilitate the formation of electronic/excitonic crystals with a wavelength～10 nm or smaller.Interestingly,the finite 2D screening from the atomically thin material can enhance the inter-site dipolar interaction, thus helping to realize the excitonic crystal.Our work can help to understand the requirement of the intriguing electronic/excitonic crystal phase in 2D semiconductor moir´e patterns.

Acknowledgements

H.Y.acknowledges support by the National Natural Science Foundation of China(Grant No.12274477)and the Department of Science and Technology of Guangdong Province of China(Grant No.2019QN01X061).