Construction of semisimple categoryover generalized Yetter-Drinfeld modules
Zhang Xiaohui Wang Shuanhong
(Department of Mathematics, Southeast University, Nanjing 211189, China)
In 2007, Panaite and Staic[1]introduced the notion of generalized Yetter-Drinfeld modules which covered both Yetter-Drinfeld modules and anti-Yetter-Drinfeld modules. Liu and Wang[2]studied the notion of generalized weak Yetter-Drinfeld modules and made the category ofHWYDH(α,β) into a braided T-category[3]. The fusion category[4-6]plays an important role in classifying the semisimple Hopf algebra. The semisimple category is the first step to construct a fusion category. In this paper, we discuss the following question: how to make the category of generalized Yetter-Drinfeld modulesHYDH(α,β) into a semisimple category.
Throughout this paper, we assume thatHis a Hopf algebra[7-8]with a bijective antipode over a fieldk. Denote the set of all the automorphisms ofHby AutHopf(H). Letα,β∈AutHopf(H).
1 Preliminaries
Definition1For anyα,β∈AutHopf(H), a (α,β)-Yetter-Drinfeld module is ak-moduleM, such thatMis a leftH-module (with notationh⊗m|→h·m) and a rightH-comodule (with notationm|→m(0)⊗m(1)) with the following compatibility condition:
ρ(h·m)=h2·m(0)⊗β(h3)m(1)α(S-1(h1))
for allh∈Handm∈M. The category of (α,β)-Yetter-Drinfeld modules andH-linearH-colinear maps is denoted byHYDH(α,β).
Define the category of the generalized Yetter-Drinfeld module YD(H) as the disjoint union of allHYDH(α,β).
Definition2Suppose thatM∈YD(H), thenMis called simple if it has no proper subobjects. A direct sum of simple objects is called semisimple. If every objectM∈YD(H) is semisimple, we call the category YD(H) semisimple.
2 Making YD(H) into semisimple
Lemma1[1]Suppose thatM∈HYDH(α,β) andN∈HYDH(γ,δ), thenM⊗N∈HYDH(αγ,δγ-1βγ) with the following structures
h·(m⊗n)=γ(h1)·m⊗γ-1βγ(h2)·n
m⊗n|→(m⊗n)(0)⊗(m⊗n)(1)=(m(0)⊗n(0))⊗n(1)m(1)
Lemma2Ifkis a commutative ring,His a commutative Hopf algebra overk,M∈HYDH(α,β),N∈HYDH(γ,δ), andMis a finitely generated projectiveH-module, then
1)Hhom(M,N) is anH-comodule, andHhomH(M,N)=Hhom(M,N)coH, where theH-coaction is given byρ(f)(m)=f0(m)⊗f1f(m0)0⊗f(m0)1S(m1).
2)Hhom(M,N)∈HYDH(α,β), where theH-action is given by (h·f)(m)hf(m)=f(h·m).
Proof1) Define a mapπ:Hhom(M,N)→Hhom(M,N⊗H) byπ(f)(m)=f(m0)0⊗f(m0)1S(m1).
For anym∈M,h∈H, we have
π(f)(h·m)=f((h·m)0)0⊗f((h·m)0)1S((h·m)1)=
f(h2·m0)0⊗f(h2·m0)1S(β(h3)m1α(S-1(h1)))=
h·f(m0)0⊗β(h3)β(S(h4))f(m0)1α(S-1(h2))·
α(h1)S(m1)=h·f(m0)0⊗f(m0)1S(m1)=
h·(π(f))(m))
Thus,πis well defined. SinceMis a finitely generated projectiveH-module, we haveHhom(M,N⊗H)≅Hhom(M,N)⊗H. So we obtain a map:
ρ:Hhom(M,N)→Hhom(M,N)⊗H
such thatρ(f)(m)=f(m0)0⊗f(m0)1S(m1), andHhom(M,N)∈MH.
Now for anyf∈Hhom(M,N), iffisH-colinear, then
ρ(f)(m)=f(m0)0⊗f(m0)1S(m1)=
f(m0)⊗m1S(m2)=f(m)⊗1=(f⊗1)(m)
Sofis coinvariant. Conversely, takef∈Hhom(M,N)coH, then we have
ρN(f(m))=f(m0)0⊗f(m0)1ε(m1)=
f(m0)0⊗f(m0)1S(m1)m2=f(m0)⊗m1
for anym∈M, andfisH-linear. Thus,HhomH(M,N)=Hhom(M,N)coH.
2) For anym∈M,h∈H, we have
((h·f)0⊗(h·f)1)(m)=(h·f(m0))0⊗
(h·f(m0))1S(m1)=h2·f(m0)0⊗
β(h3)f(m0)1α(S-1(h1))S(m1)=
(h2·f0⊗β(h3)f1α(S-1(h1)))(m)
Lemma3LetVbe ak-module andNbe anH-module, then
1)Hhom(H⊗V,N) and hom(V,N) are isomorphic ask-modules, where the bijection is given byθ:Hhom(H⊗V,N)→hom(V,N),θ(f)(v)=f(1⊗v).
2) IfVis a projectivek-module, thenH⊗Vis a projectiveH-module.
Furthermore, ifV∈MH, thenH⊗Vis an object ofHYDH(α,β) via
h·(h′⊗v)=hh′⊗v
ρ(h⊗v)=h2⊗v0⊗β(h3)v1α(S-1(h1))
Similar to Lemma 2, we can obtain the following lemmas.
Lemma4LetV∈MHis a finitely generated projectivek-module. Then for anyH-comoduleN, we have hom(V,N)∈MH, where theH-coaction is given byρ(g)(v)=g(v0)0⊗g(v0)1S(v1). IfHis commutative, then for anyN∈HYDH(α,β), we can getHhom(H⊗V,N)∈HYDH(α,β).
Lemma5Suppose thatHis commutative, andN∈HYDH(α,β).
1) IfV∈MHis a finitely generated projectivek-module, thenHhom(H⊗V,N) and hom(V,N) are isomorphic asH-comodules.
2) Ifkis a field,Vis a finite-dimensionalk-space and a projective rightH-comodule, thenH⊗Vis a projective object inHYDH(α,β).
Proof1) It is straightforward.
2) Obviously, we have
HhomH(H⊗V,N)≅Hhom(H⊗V,N)coH≅
hom(V,N)coH≅homH(V,N)
where the last isomorphism is due to the proof of Lemma 2. So the conclusion holds. From the above two lemmas, we have the following facts.
Lemma6Letkbe a field, andM∈HYDH(α,β). ThenMis a finitely generatedH-module if and only if there exists a finite dimensionalH-comoduleVand anH-linearH-colinear epimorphismπ:H⊗V→M.
LetH*be the linear dual ofH. IfM,N∈MH, then homk(M,N)∈H*Munder the followingH*-action
(h*·f)(m)=h*(f(m0)1S(m1))·f(m0)0
Lemma7Assume thatHis commutative, andM,N∈HYDH(α,β). ThenHhom(M,N) is a leftH*-submodule of homk(M,N).
Furthermore,M∈H*Mis called rational if the leftH*-action onMis induced by a rightH-coaction onM.
Proposition1Suppose thatHis commutative,kis a field,M,N∈HYDH(α,β), andMis a finitely generatedH-module. ThenHhom(M,N)∈HYDH(α,β).
ProofBy Lemma 6, there exists a finite dimensionalH-comoduleVand anH-linearH-colinear epimorphismπ:H⊗V→M. So we obtain an injectivek-linear mapHhom(π,N):Hhom(M,N)→Hhom(H⊗V,N). For anyφ∈H*,v∈V,h∈H,f∈Hhom(M,N), we haveπ(h⊗v)=h·v,ρ(1⊗v)=1⊗v0⊗v1, and
((φ·f)∘π)(1⊗v)=(φ·f)(v)=
φ(f(v0)1S(v1))f(v0)0=
φ(f(π(1⊗v0))1S(v1))f(π(1⊗v0))0=
φ(f(π(1⊗v)0)1S(1⊗v)1)f(π(1⊗v)0)0=
(φ·(f∘π))(1⊗v)
It follows thatHhom(π,N) isH*-linear. Then by Lemma 2,Hhom(H⊗V,N) is anH-comodule, and, therefore, a rationalH*-module. ThusHhom(M,N) is a rationalH*-submodule ofHhom(H⊗V,N). This means thatHhom(M,N) is anH-comodule. Then we obtainHhom(M,N)∈HYDH(α,β) by Lemma 2.
We say thatHYDH(α,β) satisfies the exact condition if the following property holds: ifM∈HYDH(α,β) is a finitely generatedH-module, then the functorHhom(M,_):HYDH(α,β)→HYDH(α,β) is exact.
By Proposition 1, ifHis commutative andMis a finitely generatedH-module, we haveHhom(M,N)∈HYDH(α,β) for anyN∈HYDH(α,β). ObviouslyHYDH(α,β) satisfies the exact condition ifHis semisimple.
Proposition2Assume thatHis commutative, andHYDH(α,β) satisfies the exact condition and the functor (-)coH:HYDH(α,β)→kMis exact. Then any finitely generatedH-moduleM∈HYDH(α,β) is a projective object.
Proofwe haveHhomH(M,_)≅Hhom(M,_)coH=(-)coH∘Hhom(M,_) which implies thatHhomH(M,_) is also an exact functor.
Proposition3Under the same condition of Proposition 2, suppose thatHis noetherian. Then any finitely generatedH-moduleM∈HYDH(α,β) is a direct sum of a family of simple subobjects which are also finitely generated asH-modules inHYDH(α,β).
ProofAssume thatNis a subobject ofM. ThenNandM/Nare finitely generatedH-modules sinceHis noetherian. Furthermore,NandM/Nare projective objects. So we have a split exact sequence inHYDH(α,β): 0→N→M→M/N→0.
Thus the conclusion holds.
TakeM∈HYDH(α,β) and anH-subcomoduleVofM. We set
whereIis a finite set. ThenHVis a subobject ofMinHYDH(α,β) via:
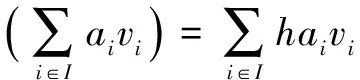
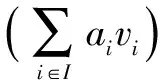
Theorem1LetHbe commutative and noetherian,HYDH(α,β) satisfies the exact condition and the functor (-)coH:HYDH(α,β)→kMis exact. Then everyM∈HYDH(α,β) is a direct sum of a family of simple subobjects ofMwhich are finitely generated asH-modules inHYDH(α,β). Therefore,HYDH(α,β) is a semisimple category.
ProofFor anym∈M,mbelongs to a finite dimensionalH-subcomoduleVmofM. ThenVmis a finitely generatedH-module. By Proposition 3,Vmis a direct sum of a family of simple subobjects which are finitely generated. LetΩbe the set of all direct sumsN=⨁i∈INiwhere everyNiis both a finitely generatedH-module and a simple subobject ofMinHYDH(α,β). Then the sum of two elements inΩis also an object inΩ. ThusΩcontains a maximal elementM′ through Zorn’s Lemma. For anym∈M, we havem∈HVm∈Ω. This means thatHVm+M′=M′. SoM=M′. Thus, the conclusion holds.
Corollary1LetHbe commutative and noetherian (particularly finite dimensional), semisimple and cosemisimple. Then eachM∈HYDH(α,β) is a direct sum of a family of simple subobjects ofMwhich are finitely generated asH-modules inHYDH(α,β). HenceHYDH(α,β) is a semisimple category.
ProofSinceHis cosemisimple, the functor (-)coH:MH→kMis exact. Thus (-)coH:HYDH(α,β)→kMis exact. Furthermore, the semisimplicity implies thatHYDH(α,β) satisfies the exact condition. Then by Theorem 1, the conclusion holds.
Theorem2As the disjoint union of allHYDH(α,β), the category of the generalized Yetter-Drinfeld modules YD(H) is also semisimple.
[1]Panaite F, Staic M D. Generalized (anti) Yetter-Drinfeld modules as components of a braided T-category [J].IsraelJMath, 2007,158(1): 349-365.
[2]Liu L, Wang S H. Constructing new braided T-categories over weak Hopf algebras [J].ApplCategorStruct, 2010,18(4): 431-459.
[3]Turaev V.Homotopyquantumfieldtheory[M]. Bloomington: European Mathematical Society, 2010.
[4]Etingof P, Nikshych D, Ostrik V. On fusion categories [J].AnnalsofMathematics, 2005,162(2): 581-642.
[5]Drinfeld V, Gelaki S, Nikshych D, Ostrik V. On braided fusion categories Ⅰ [J].SelectaMathematica, 2010,16(1): 1-119.
[6]Naidu D, Rowell E. A finiteness property for braided fusion categories [J].AlgebrRepresentTheor, 2011,14(5): 837-855.
[7]Sweedler M.Hopfalgebras[M]. New York: Benjamin, 1969.
[8]Montgomery S.Hopfalgebrasandtheiractionsonrings[M]. Rhode Island: American Mathematical Society, 1993.
 Journal of Southeast University(English Edition)2013年4期
Journal of Southeast University(English Edition)2013年4期
- Journal of Southeast University(English Edition)的其它文章
- Optimal decision thresholdfor soft decision cooperative spectrum sensing
- Two-level Bregmanized method for image interpolation with graph regularized sparse coding
- Exponential stabilization of distributed parameter switched systems under dwell time constraints
- Direct linear discriminant analysis based on column pivoting QR decomposition and economic SVD
- RBF neural network regression modelbased on fuzzy observations
- Design and analysis of dual-mode structure repetitive control based hybrid current regulation scheme for active power filters
